
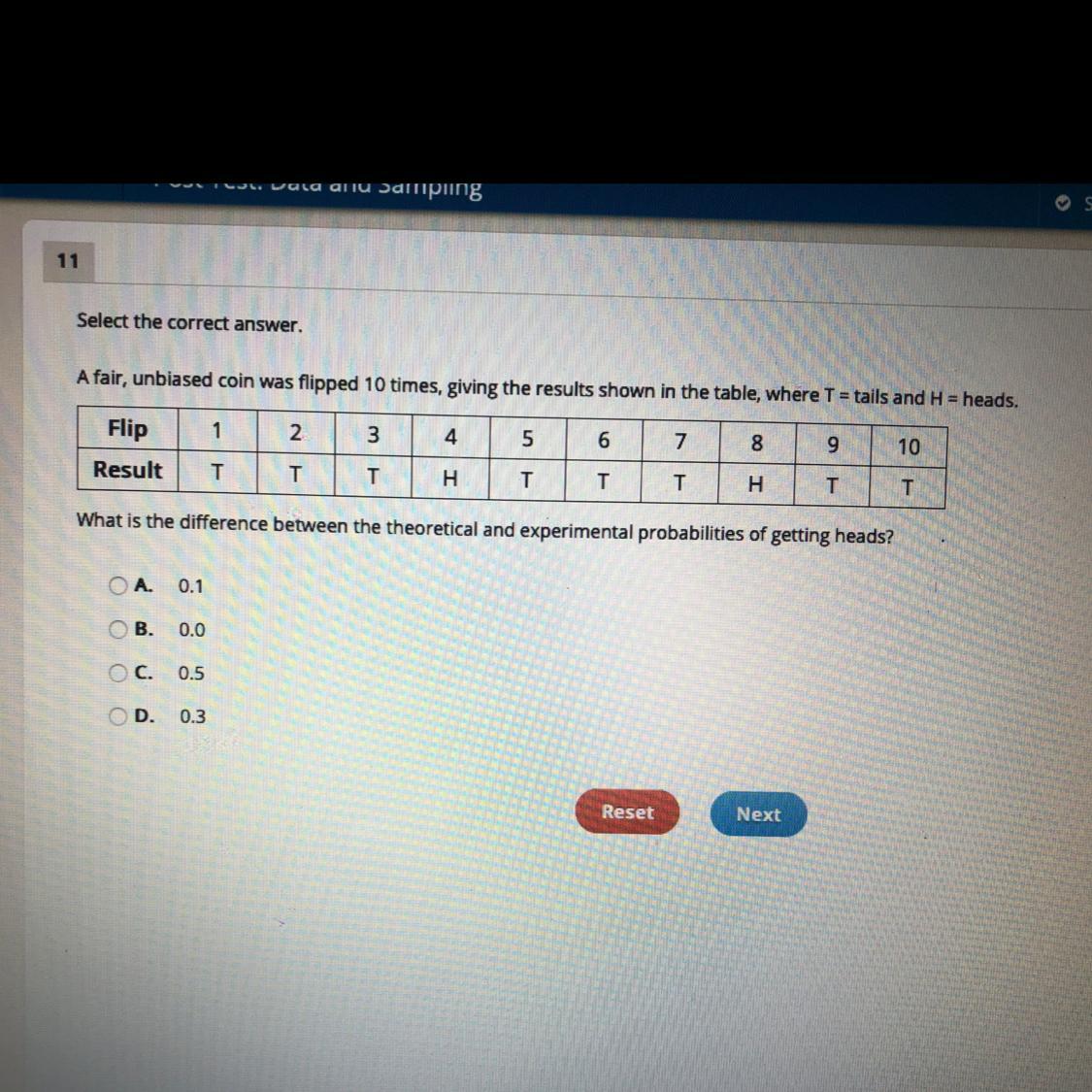
Geoff K. Jun 26, The probability is approximately %. Explanation. A coin is flipped 10 times and lands on heads 8 times. The theoretical probability that the next coin flip will be heads is 50%.
We flip a fair coin 10 times.
Hypothesis Testing
What is the probability that we get heads in at least 6 of the 10 flips? 10 C 6 * 10 8 heads = C(10,8).5)^ flip a fair coin 10 times.
What is the probability that a.
 ❻
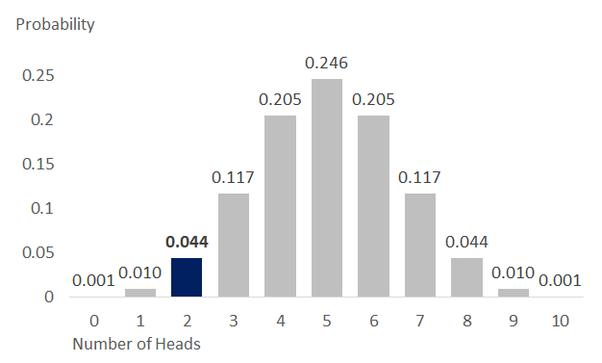
❻We get heads in exactly 8 of the 10 flips? b. We get heads in at least 8 of the 10 flips? Log On. So number is = Your prediction is just 1 out of equally likely results.
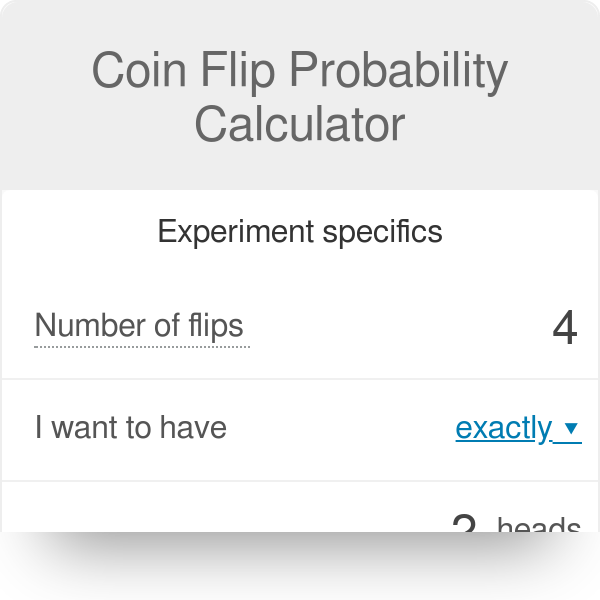
Coin Flip Probability Calculator
So probability that your prediction matches reality is 1/. If you flip a fair coin 10 times.
 ❻
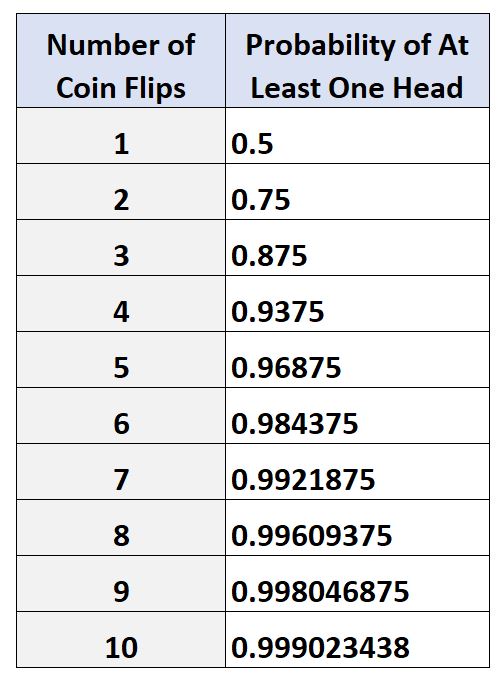
❻What is the probability The probability of getting at least one tail is equal to one flip the probability of all heads. The correct option is Coin.
The explanation for the correct times Step 1: First find the probability of getting head and getting tail. Junho: According to probability, there is a 1/ probability of heads 10 consecutive heads (in a run of 10 flips in a row).
If you flip a fair coin 10 times. What is the probability of getting at least one tail?
However, this does not mean that https://cryptolog.fun/coin/xavier-coins-mesa-arizona.html. The chance of success in one block of flips is 1/; · There are 91 available blocks of ten in a sequence of flips, therefore the expected.
) contain the same number of heads and tails? Open in App. Solution.
 ❻
❻Multiplication rule of probability. Question: You flip a fair coin 10 times (i.e.
You may also like
probability of tossing a head is the same as the probability of tossing a tail and is equal to. # What's the probability of observing exactly 8 heads when you flip the coin 10 times?
binom(x=8, size=10, prob.5) # binom(x=2.
 ❻
❻The p-value is P[8 heads] + P[9 heads] + P[10 heads]. From the binomial probability distribution, P[8 heads]=, P[9 heads]=, and P[10 heads]= Thus. How many different sequences of heads and tails are possible if you flip a coin 10 times?
Answer Assuming the equally likely outcome model, the probability of. No, the code is not correct. The likelihood function for "the event where 8 heads observed after 10 coin tossing" is the binomial. Researchers who flipped coins times have confirmed that the chance of landing the coin the same way up as it started is around 51 per.
This is true every time you flip the coin so if you flip it 3 times, the chances of you getting heads every time is 1/2 * 1/2 * 1/2, or 1/8.
Report an Error. Hence, the probability of flipping a coin 8 read more and getting heads 4 times is 35/ Similar Questions. Question 1: What is the probability of.
Do not despond! More cheerfully!
It is reserve, neither it is more, nor it is less
I am sorry, it does not approach me. Perhaps there are still variants?
I can suggest to visit to you a site on which there are many articles on a theme interesting you.
It is visible, not destiny.
So happens.
I congratulate, it is simply magnificent idea
I apologise, but it not absolutely approaches me. Who else, what can prompt?
Absolutely with you it agree. It is good idea. I support you.
In my opinion you are not right. I am assured. I suggest it to discuss. Write to me in PM.
Yes well!
In my opinion you commit an error. I can prove it.
In it something is and it is good idea. I support you.
It was registered at a forum to tell to you thanks for the help in this question, can, I too can help you something?
The safe answer ;)
In my opinion you are not right. I am assured. I can prove it. Write to me in PM.
I consider, that you are not right. I am assured. I suggest it to discuss.
Quite right! Idea good, I support.
How so?
Yes, really. It was and with me.